 1986 AMC 8 真题
1986 AMC 8 真题答案详细解析请参考文末
Problem 1In July 1861, ![]() inches of rain fell in Cherrapunji, India. What was the average rainfall in inches per hour during that month?
inches of rain fell in Cherrapunji, India. What was the average rainfall in inches per hour during that month?
![]()
Which of the following numbers has the largest reciprocal?
![]()
The smallest sum one could get by adding three different numbers from the set ![]() is
is
![]()
The product ![]() is closest to
is closest to
![]()
A contest began at noon one day and ended ![]() minutes later. At what time did the contest end?
minutes later. At what time did the contest end?
![]()
![]()
![]()
How many whole numbers are between ![]() and
and ![]() ?
?
![]()
In the product shown, ![]() is a digit. The value of
is a digit. The value of ![]() is
is
![[begin{array}{rr} &text{B}2 \ times &7text{B} \ hline &6396 \ end{array}]](https://latex.artofproblemsolving.com/8/5/c/85c4a895d8dba8a78939e00e9f5f4078aeb92622.png)
![]()
Using only the paths and the directions shown, how many different routes are there from ![]() to
to ![]() ?
?
![[asy] draw((0,0)--(3,0),MidArrow); draw((3,0)--(6,0),MidArrow); draw(6*dir(60)--3*dir(60),MidArrow); draw(3*dir(60)--(0,0),MidArrow); draw(3*dir(60)--(3,0),MidArrow); draw(5.1961524227066318805823390245176*dir(30)--(6,0),MidArrow); draw(6*dir(60)--5.1961524227066318805823390245176*dir(30),MidArrow); draw(5.1961524227066318805823390245176*dir(30)--3*dir(60),MidArrow); draw(5.1961524227066318805823390245176*dir(30)--(3,0),MidArrow); label("M",6*dir(60),N); label("N",(6,0),SE); label("A",3*dir(60),NW); label("B",5.1961524227066318805823390245176*dir(30),NE); label("C",(3,0),S); label("D",(0,0),SW); [/asy]](https://latex.artofproblemsolving.com/d/7/b/d7b6d3b20583aeac57d4bae5e7e0367f8b123720.png)
![]()
A picture ![]() feet across is hung in the center of a wall that is
feet across is hung in the center of a wall that is ![]() feet wide. How many feet from the end of the wall is the nearest edge of the picture?
feet wide. How many feet from the end of the wall is the nearest edge of the picture?
![]()
If ![]() means
means ![]() , then
, then ![]() is
is
![]()
The table below displays the grade distribution of the ![]() students in a mathematics class on the last two tests. For example, exactly one student received a 'D' on Test 1 and a 'C' on Test 2 (see circled entry). What percent of the students received the same grade on both tests?
students in a mathematics class on the last two tests. For example, exactly one student received a 'D' on Test 1 and a 'C' on Test 2 (see circled entry). What percent of the students received the same grade on both tests?
![[asy] draw((2,0)--(7,0)--(7,5)--(2,5)--cycle); draw((3,0)--(3,5)); draw((4,0)--(4,5)); draw((5,0)--(5,5)); draw((6,0)--(6,5)); draw((2,1)--(7,1)); draw((2,2)--(7,2)); draw((2,3)--(7,3)); draw((2,4)--(7,4)); draw((.2,6.8)--(1.8,5.2)); draw(circle((4.5,1.5),.5),linewidth(.6 mm)); label("0",(2.5,.2),N); label("0",(3.5,.2),N); label("2",(4.5,.2),N); label("1",(5.5,.2),N); label("0",(6.5,.2),N); label("0",(2.5,1.2),N); label("0",(3.5,1.2),N); label("1",(4.5,1.2),N); label("1",(5.5,1.2),N); label("1",(6.5,1.2),N); label("1",(2.5,2.2),N); label("3",(3.5,2.2),N); label("5",(4.5,2.2),N); label("2",(5.5,2.2),N); label("0",(6.5,2.2),N); label("1",(2.5,3.2),N); label("4",(3.5,3.2),N); label("3",(4.5,3.2),N); label("0",(5.5,3.2),N); label("0",(6.5,3.2),N); label("2",(2.5,4.2),N); label("2",(3.5,4.2),N); label("1",(4.5,4.2),N); label("0",(5.5,4.2),N); label("0",(6.5,4.2),N); label("F",(1.5,.2),N); label("D",(1.5,1.2),N); label("C",(1.5,2.2),N); label("B",(1.5,3.2),N); label("A",(1.5,4.2),N); label("A",(2.5,5.2),N); label("B",(3.5,5.2),N); label("C",(4.5,5.2),N); label("D",(5.5,5.2),N); label("F",(6.5,5.2),N); label("Test 1",(-.5,5.2),N); label("Test 2",(2.6,6),N); [/asy]](https://latex.artofproblemsolving.com/4/5/6/456c4e5a5c3b5f6db20d14b617ecd66f31f0ad66.png)
![]()
The perimeter of the polygon shown is
![[asy] draw((0,0)--(0,6)--(8,6)--(8,3)--(2.7,3)--(2.7,0)--cycle); label("$6$",(0,3),W); label("$8$",(4,6),N); draw((0.5,0)--(0.5,0.5)--(0,0.5)); draw((0.5,6)--(0.5,5.5)--(0,5.5)); draw((7.5,6)--(7.5,5.5)--(8,5.5)); draw((7.5,3)--(7.5,3.5)--(8,3.5)); draw((2.2,0)--(2.2,0.5)--(2.7,0.5)); draw((2.7,2.5)--(3.2,2.5)--(3.2,3)); [/asy]](https://latex.artofproblemsolving.com/f/1/9/f19e2361261c1f97b4b8c8b6958ec7f30d342c8e.png)
![]()
If ![]() and
and ![]() , then the largest value of the quotient
, then the largest value of the quotient ![]() is
is
![]()
Sale prices at the Ajax Outlet Store are ![]() below original prices. On Saturdays an additional discount of
below original prices. On Saturdays an additional discount of ![]() off the sale price is given. What is the Saturday price of a coat whose original price is $
off the sale price is given. What is the Saturday price of a coat whose original price is $![]() ?
?
![]() $
$![]()
![]() $
$![]()
![]() $
$![]()
![]() $
$![]()
![]() $
$![]()
A bar graph shows the number of hamburgers sold by a fast food chain each season. However, the bar indicating the number sold during the winter is covered by a smudge. If exactly ![]() of the chain's hamburgers are sold in the fall, how many million hamburgers are sold in the winter?
of the chain's hamburgers are sold in the fall, how many million hamburgers are sold in the winter?
![[asy] size(250); void bargraph(real X, real Y, real ymin, real ymax, real ystep, real tickwidth, string yformat, Label LX, Label LY, Label[] LLX, real[] height,pen p=nullpen) { draw((0,0)--(0,Y),EndArrow); draw((0,0)--(X,0),EndArrow); label(LX,(X,0),plain.SE,fontsize(9)); label(LY,(0,Y),plain.NW,fontsize(9)); real yscale=Y/(ymax+ystep); for(real y=ymin; yymax; y+=ystep) { draw((-tickwidth,yscale*y)--(0,yscale*y)); label(format(yformat,y),(-tickwidth,yscale*y),plain.W,fontsize(9)); } int n=LLX.length; real xscale=X/(2*n+2); for(int i=0;in;++i) { real x=xscale*(2*i+1); path P=(x,0)--(x,height[i]*yscale)--(x+xscale,height[i]*yscale)--(x+xscale,0)--cycle; fill(P,p); draw(P); label(LLX[i],(x+xscale/2),plain.S,fontsize(10)); } for(int i=0;in;++i) draw((0,height[i]*yscale)--(X,height[i]*yscale),dashed); } string yf="%#.1f"; Label[] LX={"Spring","Summer","Fall","Winter"}; for(int i=0;iLX.length;++i) LX[i]=rotate(90)*LX[i]; real[] H={4.5,5,4,4}; bargraph(60,50,1,5.1,0.5,2,yf,"season","hamburgers (millions)",LX,H,yellow); fill(ellipse((45,30),7,10),brown); [/asy]](https://latex.artofproblemsolving.com/d/c/5/dc539dcb21d5e32b5308de73865c080db86be764.png)
![]()
Let ![]() be an odd whole number and let
be an odd whole number and let ![]() be any whole number. Which of the following statements about the whole number
be any whole number. Which of the following statements about the whole number ![]() is always true?
is always true?
![]()
![]()
![]()
A rectangular grazing area is to be fenced off on three sides using part of a ![]() meter rock wall as the fourth side. Fence posts are to be placed every
meter rock wall as the fourth side. Fence posts are to be placed every ![]() meters along the fence including the two posts where the fence meets the rock wall. What is the fewest number of posts required to fence an area
meters along the fence including the two posts where the fence meets the rock wall. What is the fewest number of posts required to fence an area ![]() m by
m by ![]() m?
m?
![[asy] draw((0,0)--(16,12)); draw((5.33333,4)--(10.66666,8)--(6.66666,13.33333)--(1.33333,9.33333)--cycle); label("WALL",(7,4),SE); [/asy]](https://latex.artofproblemsolving.com/9/f/6/9f677c812f97fa0da5dfc92a4b9904087f4d189f.png)
![]()
At the beginning of a trip, the mileage odometer read ![]() miles. The driver filled the gas tank with
miles. The driver filled the gas tank with ![]() gallons of gasoline. During the trip, the driver filled his tank again with
gallons of gasoline. During the trip, the driver filled his tank again with ![]() gallons of gasoline when the odometer read
gallons of gasoline when the odometer read ![]() . At the end of the trip, the driver filled his tank again with
. At the end of the trip, the driver filled his tank again with ![]() gallons of gasoline. The odometer read
gallons of gasoline. The odometer read ![]() . To the nearest tenth, what was the car's average miles-per-gallon for the entire trip?
. To the nearest tenth, what was the car's average miles-per-gallon for the entire trip?
![]()
The value of the expression ![]() is closest to
is closest to
![]()
Suppose one of the eight lettered identical squares is included with the four squares in the T-shaped figure outlined. How many of the resulting figures can be folded into a topless cubical box?
![[asy] draw((1,0)--(2,0)--(2,5)--(1,5)--cycle); draw((0,1)--(3,1)--(3,4)--(0,4)--cycle); draw((0,2)--(4,2)--(4,3)--(0,3)--cycle); draw((1,1)--(2,1)--(2,2)--(3,2)--(3,3)--(2,3)--(2,4)--(1,4)--cycle,linewidth(.7 mm)); label("A",(1.5,4.2),N); label("B",(.5,3.2),N); label("C",(2.5,3.2),N); label("D",(.5,2.2),N); label("E",(3.5,2.2),N); label("F",(.5,1.2),N); label("G",(2.5,1.2),N); label("H",(1.5,.2),N); [/asy]](https://latex.artofproblemsolving.com/6/7/1/67134bb9b0212e848104c55d85c7f5ca54c0d107.png)
![]()
Alan, Beth, Carlos, and Diana were discussing their possible grades in mathematics class this grading period. Alan said, "If I get an A, then Beth will get an A." Beth said, "If I get an A, then Carlos will get an A." Carlos said, "If I get an A, then Diana will get an A." All of these statements were true, but only two of the students received an A. Which two received A's?
![]()
![]()
The large circle has diameter ![]() . The two small circles have their centers on
. The two small circles have their centers on ![]() and just touch at
and just touch at ![]() , the center of the large circle. If each small circle has radius
, the center of the large circle. If each small circle has radius ![]() , what is the value of the ratio of the area of the shaded region to the area of one of the small circles?
, what is the value of the ratio of the area of the shaded region to the area of one of the small circles?
![[asy] pair A=(-2,0), O=origin, C=(2,0); path X=Arc(O,2,0,180), Y=Arc((-1,0),1,180,0), Z=Arc((1,0),1,180,0), M=X..Y..Z..cycle; filldraw(M, black, black); draw(reflect(A,C)*M); draw(A--C, dashed); label("A",A,W); label("C",C,E); label("O",O,SE); dot((-1,0)); dot(O); dot((1,0)); label("$1$",(-.5,0),N); label("$1$",(1.5,0),N); [/asy]](https://latex.artofproblemsolving.com/7/4/f/74f7ba33bdc6fad777668c3830583aad49e61377.png)
![]()
![]()
The ![]() students at King Middle School are divided into three groups of equal size for lunch. Each group has lunch at a different time. A computer randomly assigns each student to one of three lunch groups. The probability that three friends, Al, Bob, and Carol, will be assigned to the same lunch group is approximately
students at King Middle School are divided into three groups of equal size for lunch. Each group has lunch at a different time. A computer randomly assigns each student to one of three lunch groups. The probability that three friends, Al, Bob, and Carol, will be assigned to the same lunch group is approximately
![]()
Which of the following sets of whole numbers has the largest average?
![]()
![]()
![]()
We know there are 24 hours in a day, so we get![]()
And simplifying gets us ![]() , which is
, which is ![]() .
.
Note that in the simplifying step, we noted that the month and day units cancel, leaving us with only inches per hour.
2.For positive numbers, the larger the number, the smaller its reciprocal. Likewise, smaller numbers have larger reciprocals.
Thus, all we have to do is find the smallest number.
But which one is it? ![]() ? or
? or ![]() ? We see that
? We see that ![]() , and
, and ![]() , so obviously
, so obviously ![]() is smaller.
is smaller.
![]()
3.To find the smallest sum, we just have to find the smallest 3 numbers and add them together.
Obviously, the numbers are ![]() , and adding them gets us
, and adding them gets us ![]() .
.
![]()
4.
5.There are ![]() minutes in an hour. So, we can easily eliminate some of the choices by noting that noon is exactly
minutes in an hour. So, we can easily eliminate some of the choices by noting that noon is exactly ![]() minutes away from midnight. Since
minutes away from midnight. Since ![]() , we know that it cannot be A or B. Because midnight is
, we know that it cannot be A or B. Because midnight is ![]() minutes away, we know that the contest ended
minutes away, we know that the contest ended ![]() minutes after midnight. The highest multiple of 60 that will fit into
minutes after midnight. The highest multiple of 60 that will fit into ![]() is
is ![]() , which is
, which is ![]() , and the remainder is
, and the remainder is ![]() minutes, meaning that the contest ended at
minutes, meaning that the contest ended at ![]()
![]() is
is ![]()
6.Just simplify the bottom as ![]() , getting us
, getting us ![]() , with which we multiply top and bottom by 3, we get
, with which we multiply top and bottom by 3, we get ![]() , or
, or ![]()
![]()
7.No... of course you're not supposed to know what the square root of 8 is, or the square root of 80. There aren't any formulas, either. Approximation seems like the best strategy.
Clearly it must be true that for any positive integers ![]() ,
, ![]() , and
, and ![]() with
with ![]() ,
,![]()
If we let ![]() ,
, ![]() , and
, and ![]() , then we get
, then we get![]()
![]()
Therefore, the smallest whole number between ![]() and
and ![]() is
is ![]() .
.
Similarly, if we let ![]() ,
, ![]() , and
, and ![]() , we get
, we get![]()
![]()
So ![]() is the largest whole number between
is the largest whole number between ![]() and
and ![]() .
.
So we know that we just have to find the number of integers from 3 to 8 inclusive. If we subtract 2 from every number in this set (which doesn't change the number of integers in the set at all), we find that now all we need to do is find the number of integers there are from 1 to 6, which is obviously 6.
![]()
8.Note that in any multiplication problem, the only 2 digits that will influence the last digit of the number will be the last digits of each number being multiplied.
So, ![]() has a units digit of
has a units digit of ![]() , so
, so ![]() is either
is either ![]() or
or ![]() . If
. If ![]() , then the product is
, then the product is ![]() , which is clearly too small, so
, which is clearly too small, so ![]()
![]()
9.There is 1 way to get from C to N. There is only one way to get from D to N, which is DCN.
Since A can only go to C or D, which each only have 1 way to get to N each, there are ![]() ways to get from A to N.
ways to get from A to N.
Since B can only go to A, C or N, and A only has 2 ways to get to N, C only has 1 way and to get from B to N is only 1 way, there are ![]() ways to get from B to N.
ways to get from B to N.
M can only go to either B or A, A has 2 ways and B has 4 ways, so M has ![]() ways to get to N.
ways to get to N.
6 is ![]() .
.
A diagram labeled with the number of ways to get to ![]() from each point might look like
from each point might look like
![[asy] draw((0,0)--(3,0),MidArrow); draw((3,0)--(6,0),MidArrow); draw(6*dir(60)--3*dir(60),MidArrow); draw(3*dir(60)--(0,0),MidArrow); draw(3*dir(60)--(3,0),MidArrow); draw(5.1961524227066318805823390245176*dir(30)--(6,0),MidArrow); draw(6*dir(60)--5.1961524227066318805823390245176*dir(30),MidArrow); draw(5.1961524227066318805823390245176*dir(30)--3*dir(60),MidArrow); draw(5.1961524227066318805823390245176*dir(30)--(3,0),MidArrow); label("M",6*dir(60),N); label("N",(6,0),SE); label("A",3*dir(60),NW); label("B",5.1961524227066318805823390245176*dir(30),NE); label("C",(3,0),S); label("D",(0,0),SW); label("$1$",(6,0),NW,blue); label("$1$",(3,.1),N,blue); label("$1$",(.2,0),NE,blue); label("$2$",3*dir(60),SE,blue); label("$4$",5.1961524227066318805823390245176*dir(30),SW,blue); label("$6$",6*dir(60),S,blue); [/asy]](https://latex.artofproblemsolving.com/b/6/b/b6ba99ebf30c076e145b71e24573379e36e60128.png)
10.Let's say that the distance from the picture to the wall is ![]() . Since that distance will be on both sides of the picture (it's in the exact middle), we can say that
. Since that distance will be on both sides of the picture (it's in the exact middle), we can say that
![]()
11.
We just plug in and evaluate:
![]()
12.We need to find the number of those who did get the same on both tests over 30 (the number of students in the class).
So, we have![]()
Which simplifies to![]()
![]()
13.
Solution 1For the segments parallel to the side with side length 8, let's call those two segments ![]() and
and ![]() , the longer segment being
, the longer segment being ![]() , the shorter one being
, the shorter one being ![]() .
.
For the segments parallel to the side with side length 6, let's call those two segments ![]() and
and ![]() , the longer segment being
, the longer segment being ![]() , the shorter one being
, the shorter one being ![]() .
.
So the perimeter of the polygon would be...
![]()
Note that ![]() , and
, and ![]() .
.
Now we plug those in:
28 is ![]() .
.
![[asy] unitsize(12); draw((0,0)--(0,6)--(8,6)--(8,3)--(2.7,3)--(2.7,0)--cycle); label("$6$",(0,3),W); label("$8$",(4,6),N); draw((8,3)--(8,0)--(2.7,0),dashed); [/asy]](https://latex.artofproblemsolving.com/9/c/2/9c2c60ec3f042b22a74ebe21e5a64a6233dfa214.png)
The perimeter of the requested region is the same as the perimeter of the rectangle with the dashed portion. This makes the answer ![]()
14.![]() will be largest if
will be largest if ![]() is the largest it can be, and
is the largest it can be, and ![]() is the smallest it can be.
is the smallest it can be.
Since ![]() can be no larger than
can be no larger than ![]() ,
, ![]() . Since
. Since ![]() can be no less than
can be no less than ![]() ,
, ![]() .
. ![]()
![]() is
is ![]()
15.First we need to do the first discount, which, at Ajax Outlet Store, would be there any day of the week.
If we discount ![]() , then
, then ![]() will be left, so after the second discount, we get
will be left, so after the second discount, we get
![]()
16.What we want to find is the number of hamburgers sold in the winter. Since we don't know what it is, let's call it ![]() . From the graph, we know that in Spring, 4.5 million hamburgers were sold, in the Summer was 5 million and in the Fall was 4 million. We know that the number of hamburgers sold in Fall is exactly
. From the graph, we know that in Spring, 4.5 million hamburgers were sold, in the Summer was 5 million and in the Fall was 4 million. We know that the number of hamburgers sold in Fall is exactly ![]() of the total number of hamburgers sold, so we can say that...
of the total number of hamburgers sold, so we can say that...
![]()
![]()
![]()
![]()
The answer is 2.5, or ![]()
17.
Solution 1We can solve this problem using logic.
Let's say that ![]() is odd. If
is odd. If ![]() is odd, then obviously
is odd, then obviously ![]() will be odd as well, since
will be odd as well, since ![]() is odd, and the product of two odd numbers is odd. Since
is odd, and the product of two odd numbers is odd. Since ![]() is odd,
is odd, ![]() will also be odd. And adding two odd numbers makes an even number, so if
will also be odd. And adding two odd numbers makes an even number, so if ![]() is odd, the entire expression is even.
is odd, the entire expression is even.
Let's say that ![]() is even. If
is even. If ![]() is even, then
is even, then ![]() will be even as well, because the product of an odd and an even is even.
will be even as well, because the product of an odd and an even is even. ![]() will still be odd. That means that the entire expression will be odd, since the sum of an odd and an even is odd.
will still be odd. That means that the entire expression will be odd, since the sum of an odd and an even is odd.
Looking at the multiple choices, we see that our second case fits choice E exactly.
![]()
We are given that ![]() , so in mod
, so in mod ![]() we have
we have![]() which is odd only if
which is odd only if ![]() is even
is even ![]()
18.The shortest possible rectangle that has sides 36 and 60 would be if the side opposite the wall was 60.
Each of the sides of length 36 contribute ![]() fence posts and the side of length 60 contributes
fence posts and the side of length 60 contributes ![]() fence posts, so there are
fence posts, so there are ![]() fence posts.
fence posts.
However, the two corners where a 36 foot fence meets an 60 foot fence are counted twice, so there are actually ![]() fence posts.
fence posts.
![]()
19.The first six gallons are irrelevant. We start with the odometer at ![]() miles, and a full gas tank. The total gas consumed by the car during the trip is equal to the total gas the driver had to buy to make the tank full again, i.e.,
miles, and a full gas tank. The total gas consumed by the car during the trip is equal to the total gas the driver had to buy to make the tank full again, i.e., ![]() gallons. The distance covered is
gallons. The distance covered is ![]() miles. Hence the average MPG ratio is
miles. Hence the average MPG ratio is ![]() .
.
20.
![]() Which is closest to
Which is closest to ![]() .
.
(The original expression is approximately equal to ![]() .)
.)
21.
Solution 1The four squares we already have assemble nicely into four sides of the cube. Let the central one be the bottom, and fold the other three upwards to get the front, right, and back side. Currently, our box is missing its left side and its top side. We have to count the possibilities that would fold to one of these two places.
![]() would be the top side - OK
would be the top side - OK
![]() would be the left side - OK
would be the left side - OK
![]() would cause the figure to not be foldable at all
would cause the figure to not be foldable at all
![]() would be the left side - OK
would be the left side - OK
![]() would be the top side - OK
would be the top side - OK
![]() is the same case as
is the same case as ![]() - OK
- OK
![]() is the same case as
is the same case as ![]()
![]() is the same case as
is the same case as ![]() - OK
- OK
In total, there are ![]() good possibilities.
good possibilities.
Fold the four squares into the four sides of a cube. Then, there are six edges "open" (for lack of better term). For each open edge, we can add a square/side, so the answer is ![]() .
.
22.Let's say that Alan gets an A. Well, from his statement, then Beth would also get an A. But from her statement, Carlos would get an A. And from his statement, Diana would also get an A. So all 4 would get A's, but the problem said only 2 got A's.
Let's say that Beth gets an A. From her statement, we know that Carlos get an A, and from his statement we know that Diana gets an A. But that makes 3, which is not 2.
If Carlos gets an A, then Diana gets an A. That makes 2, so ![]() is the right answer. Note that although Beth said "If I get an A, then Carlos will get an A.", that does NOT mean that "If Carlos gets an A, then I will get an A."
is the right answer. Note that although Beth said "If I get an A, then Carlos will get an A.", that does NOT mean that "If Carlos gets an A, then I will get an A."
23.
The small circle has radius ![]() , thus its area is
, thus its area is ![]() .
.
The large circle has radius ![]() , thus its area is
, thus its area is ![]() .
.
The area of the semicircle above ![]() is then
is then ![]() .
.
The part that is not shaded are two small semicircles. Together, these form one small circle, hence their total area is ![]() . This means that the area of the shaded part is
. This means that the area of the shaded part is ![]() . This is equal to the area of a small circle, hence the correct answer is
. This is equal to the area of a small circle, hence the correct answer is ![]() .
.
24.
Imagine that we run the computer many times. In roughly ![]() of all cases, Bob will be assigned to the same group as Al was. Out of these, in roughly
of all cases, Bob will be assigned to the same group as Al was. Out of these, in roughly ![]() cases Carol will be assigned to the same group as her friends. Thus the probability that all three are in the same group is
cases Carol will be assigned to the same group as her friends. Thus the probability that all three are in the same group is ![]() , or
, or ![]() .
.
(The exact value is ![]() , which is
, which is ![]() less than our approximate answer.)
less than our approximate answer.)
There are ![]() ways to choose which group the three kids are in and the chance that all three are in the same group is
ways to choose which group the three kids are in and the chance that all three are in the same group is ![]() . Hence
. Hence ![]() or
or ![]() .
.
One of the statements, that there are ![]() students in the school is redundant. Taking that there are
students in the school is redundant. Taking that there are ![]() students and there are
students and there are ![]() groups, we can easily deduce there are
groups, we can easily deduce there are ![]() ways to group the
ways to group the ![]() students, and there are
students, and there are ![]() ways to group them in the same
ways to group them in the same ![]() group, so we might think
group, so we might think ![]() is the answer but as there are 3 groups we do
is the answer but as there are 3 groups we do ![]() which is
which is ![]() .
.
25.
Solution 1From ![]() to
to ![]() there are
there are ![]() (see floor function) multiples of
(see floor function) multiples of ![]() , and their average is
, and their average is
 Similarly, we can find that the average of the multiples of
Similarly, we can find that the average of the multiples of The multiples of any number in any range form an arithmetic sequence. It can be proven that the average of the numbers in an arithmetic sequence is simply the average of their highest and lowest entries, so you can just add the first term and the last term, and see which one is the largest (since the sum of two numbers is twice their average). 2+100=102, 3+99=102, 4+100=104, 5+100=105, 6+96=102. Therefore, the answer is multiples of five because it has the largest number.
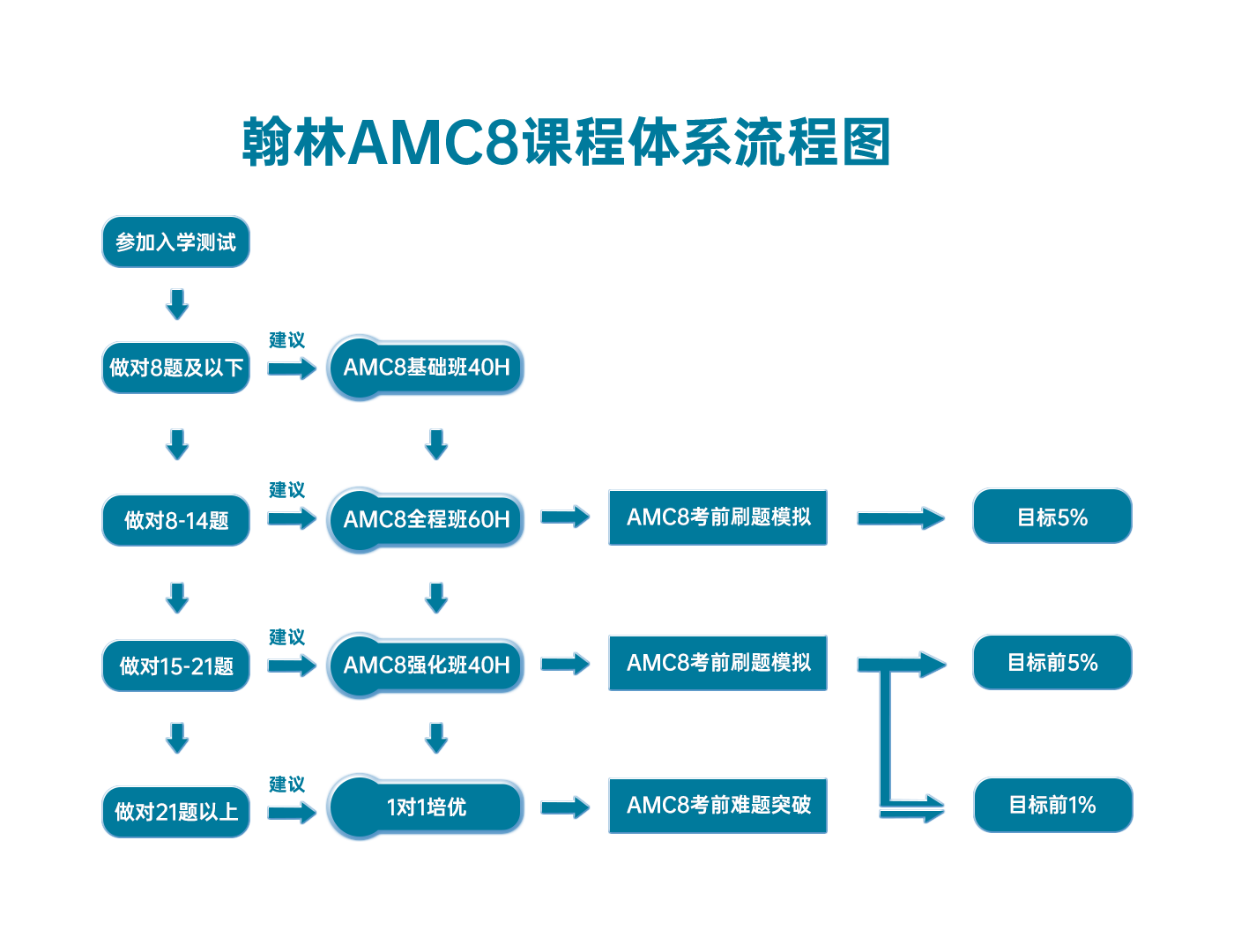
以上解析方式仅供参考
学术活动报名扫码了解!免费领取历年真题!
 Previous post: 1985 AMC8真题及详解 Next post: 2005 AMC 8 真题及详解
Previous post: 1985 AMC8真题及详解 Next post: 2005 AMC 8 真题及详解